
Kirchhoffs Circuit Law
Related Term
Kirchhoffs Current Law or KCL
states that the “total current or charge entering a junction or node is exactly equal to the charge leaving the node as it has no other place to go except to leave, as no charge is lost within the node“. In other words the algebraic sum of ALL the currents entering and leaving a node must be equal to zero, I(exiting) + I(entering) = 0. This idea by Kirchhoff is commonly known as the Conservation of Charge.
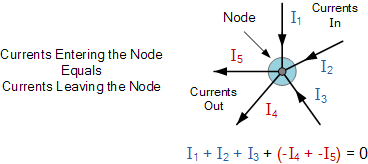
Here, the three currents entering the node, I1, I2, I3 are all positive in value and the two currents leaving the node, I4 and I5 are negative in value. Then this means we can also rewrite the equation as;
I1 + I2 + I3 – I4 – I5 = 0
The term Node in an electrical circuit generally refers to a connection or junction of two or more current carrying paths or elements such as cables and components. Also for current to flow either in or out of a node a closed circuit path must exist. We can use Kirchhoff’s current law when analysing parallel circuits.
Kirchhoffs Voltage Law
Kirchhoffs Voltage Law or KVL, states that “in any closed loop network, the total voltage around the loop is equal to the sum of all the voltage drops within the same loop” which is also equal to zero. In other words the algebraic sum of all voltages within the loop must be equal to zero. This idea by Kirchhoff is known as the Conservation of Energy.
If we consider a closed loop conventionally, if we consider all the voltage gains along the loop are positive then all the voltage drops along the loop should be considered as negative. The summation of all these voltages in a closed loop is equal to zero. Suppose n numbers of back to back connected elements form a closed loop. Among these circuit elements m number elements are voltage source and n - m number of elements drop voltage such as resistors. The voltages of sources are And voltage drops across the resistors respectively, As it is said that the voltage gain conventionally considered as positive, and voltage drops are considered as negative, the voltages along the closed loop are - Now according to Kirchhoff Voltage law, the summation of all these voltages results to zero. So accordingly Kirchhoff Second Law, ∑V = 0.